
If you need to find the surface area of a rectangular prism, you will need to measure the length, width, and height of the prism. When do you need to find the surface area of a rectangular prism? A rectangular prism has the following properties: The opposite faces of a rectangular prism are parallel, and the diagonals of a rectangular prism are perpendicular. SA = d2 + 4lw + 4wh What is a rectangular prism?Ī rectangular prism is a three-dimensional figure with six rectangular faces. If you know the diagonal of the rectangular prism, you can use that as well: Where l is the length, w is the width, and h is the height. The most common way is to use the formula: There are a few different ways that you can calculate the surface area of a rectangular prism.
#Surface area of a rectangular prism formula how to#
How to Calculate the Surface Area of a Rectangular Prism? In other words, it is the sum of the areas of its four side faces. The lateral surface area of a rectangular prism is the sum of the areas of its faces that are not bases. The surface area of a three-dimensional object is the total area of its exposed sides. Total surface area = 2lw + 2wh Lateral Surface Area of a Rectangular Prism There are two types of faces on a rectangular prism – those with dimensions l and w, and those with dimensions w and h. The area of a rectangle is length times width, so the area of a rectangular face on a rectangular prism is lw. To find the total surface area of a rectangular prism, we need to add the areas of all six faces. Surface area = 2(10 * 5 + 5 * 3 + 10 * 3) = 2(50 + 15 + 30) = 2(95) = 190 inches2 Total Surface Area of Rectangular PrismĪ rectangular prism is a three-dimensional figure with six rectangular faces. You can then use the formula above to calculate the surface area.įor example, let’s say you have a rectangular prism that is 10 inches long, 5 inches wide, and 3 inches tall. To find the surface area of a rectangular prism, you need to know the length, width, and height of the prism. Where l is the length, w is the width, and h is the height of the prism. Surface Area of Rectangular Prism Formula For example, if all measurements were in inches, then the surface area would be in square inches.

The units for measuring surface area will depend on the units used for the length, width, and height measurements. With those measurements, you can use the formula:įor example, if a rectangular prism has dimensions of length = 3, width = 4, and height = 5, then its surface area would be calculated as:Ģ(3×4 + 4×5 + 3×5) = 2(12+20+15) = 2(47) = 94 To find the surface area of a rectangular prism, you need to know the measure of two adjacent sides (length and width) and the height of the rectangle. Surface Area = 72 square feet How to find the surface area of a rectangular prismĪ rectangular prism is a solid geometric figure with six faces that are rectangles. We can plug these values into our formula to calculate the surface area.
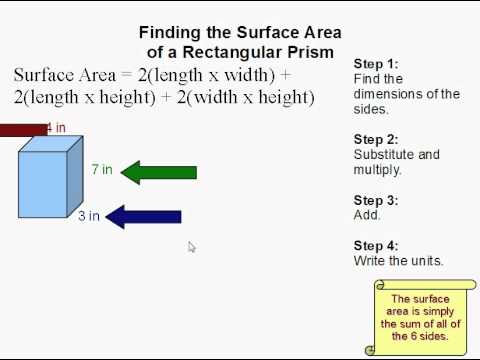
Suppose we have a rectangular prism that has dimensions of length 9 feet, width 3 feet, and height 2 feet. Let’s look at an example to see how this formula works. The surface area of a rectangular prism can be found using the formula: In the diagram below, we can see that the length (L) and width (W) are measured in units and the height (h) is measured in inches. To find the surface area of a rectangular prism, we need to know the measure of two sides and the height. The surface area of a rectangular prism is the sum of the areas of its six faces. Surface Area of Rectangular PrismĪ rectangular prism is a three-dimensional figure with six faces that are all rectangles. A rectangular prism with two dimensions will have two rectangles on each face, and so on. A rectangular prism with three dimensions will have four rectangles on each face. The number of rectangles on each face depends on how many dimensions the rectangular prism has. The surface area is the sum of the areas of all the faces. The surface area of a rectangular prism can be defined as the total area of all the faces of the prism. Surface Area of a Rectangular Prism Definitions and Examples


 0 kommentar(er)
0 kommentar(er)
